Lifeboat Foundation

Heisenberg’s uncertainty principle limits the precision with which two observables that do not commute with each other can be simultaneously measured. The Wigner-Araki-Yanase (WAY) theorem goes further. If observables A and B do not commute, and if observable A is conserved, observable B cannot be measured with arbitrary precision even if A is not measured at all. In its original 1960 formulation, the WAY theorem applied only to observables, such as spin, whose possible values are discrete and bounded. Now Yui Kuramochi of Kyushu University and Hiroyasu Tajima of the University of Electro-Communications—both in Japan—have proven that the WAY theorem also encompasses observables, such as position, that are continuous and unbounded [1]. Besides resolving the decades-long problem of how to deal with such observables, the extension will likely find practical applications in quantum optics.

The difficulty of extending the WAY theorem arose from how an unbounded observable L is represented: as an infinite-dimensional matrix with unbounded eigenvalues. To tame the problem, Kuramochi and Tajima avoided considering L directly. Instead, they looked at an exponential function of L, which forms a one-parameter unitary group. Although the exponential function is also unbounded, its spectrum of eigenvalues is contained within the complex plane’s unit circle. Thanks to that boundedness, Kuramochi and Tajima could go on to use off-the-shelf techniques from quantum information to complete their proof.

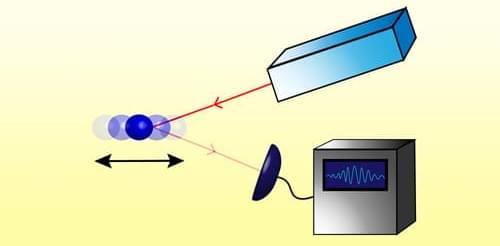
Because momentum is conserved, the extended WAY theorem implies that a particle’s position cannot be measured with arbitrary precision even if its momentum is not measured simultaneously. Similar pairs of observables crop up in quantum optics. Kuramochi and Tajima anticipate that their theorem could be useful in setting limits on the extent to which quantum versions of transmission protocols can outperform the classical ones.

Quantum scientists have discovered a phenomenon in purple bronze, a one-dimensional metal, that allows it to switch between insulating and superconducting states. This switch, triggered by minimal stimuli like heat or light, is due to ’emergent symmetry’. This groundbreaking finding, initiated by research into the metal’s magnetoresistance, could lead to the development of perfect switches in quantum devices, a potential milestone in quantum technology.

Quantum scientists have discovered a phenomenon in purple bronze that could be key to the development of a ‘perfect switch’ in quantum devices which flips between being an insulator and superconductor.

The research, led by the University of Bristol and published in Science, found these two opposing electronic states exist within purple bronze, a unique one-dimensional metal composed of individual conducting chains of atoms.

A team of researchers headed by Prof. Wang Qun at the University of Science and Technology of China, under the Chinese Academy of Sciences, has achieved a breakthrough in the theoretical understanding of vector meson spin physics, focusing on the unique properties of ϕ mesons produced during collisions between gold nuclei.

Their findings published in the journal Physical Review Letters

Physical Review Letters (PRL) is a peer-reviewed scientific journal published by the American Physical Society. It is one of the most prestigious and influential journals in physics, with a high impact factor and a reputation for publishing groundbreaking research in all areas of physics, from particle physics to condensed matter physics and beyond. PRL is known for its rigorous standards and short article format, with a maximum length of four pages, making it an important venue for rapid communication of new findings and ideas in the physics community.

Hopfions, magnetic spin structures predicted decades ago, have become a hot and challenging research topic in recent years. In a study published in Nature, the first experimental evidence is presented by a Swedish-German-Chinese research collaboration.

“Our results are important from both a fundamental and applied point of view, as a new bridge has emerged between experimental physics and abstract mathematical theory, potentially leading to hopfions finding an application in spintronics,” says Philipp Rybakov, researcher at the Department of Physics and Astronomy at Uppsala University, Sweden.

A deeper understanding of how different components of materials function is important for the development of innovative materials and future technology. The research field of spintronics, for example, which studies the spin of electrons, has opened up promising possibilities to combine the electrons’ electricity and magnetism for applications such as new electronics.

Quantum scientists have discovered a rare phenomenon that could hold the key to creating a ‘perfect switch’ in quantum devices which flips between being an insulator and a superconductor.

The research, led by the University of Bristol and published in Science, found these two opposing electronic states exist within purple bronze, a unique one-dimensional metal composed of individual conducting chains of atoms.

Tiny changes in the material, for instance, prompted by a small stimulus like heat or light, may trigger an instant transition from an insulating state with zero conductivity to a superconductor with unlimited conductivity, and vice versa. This polarized versatility, known as “emergent symmetry,” has the potential to offer an ideal On/Off switch in future quantum technology developments.

A study from an international team led by researchers from Nagoya University in Japan and the University of New Hampshire in the United States has revealed the importance of the Earth’s upper atmosphere in determining how large geomagnetic storms develop. Their findings reveal the previously underestimated importance of the Earth’s atmosphere. Understanding the factors that cause geomagnetic storms is important because they can have a direct impact on the Earth’s magnetic field such as causing unwanted currents in the power grid and disrupting radio signals and GPS. This research may help predict the storms that will have the greatest consequences.

Scientists have long known that geomagnetic storms are associated with the activities of the Sun. Hot charged particles make up the Sun’s outer layer, the one visible to us. These particles flow out of the Sun creating the ‘solar wind’, and interact with objects in space, such as the Earth. When the particles reach the magnetic field surrounding our planet, known as the magnetosphere, they interact with it. The interactions between the charged particles and magnetic fields lead to space weather, the conditions in space that can affect the Earth and technological systems such as satellites.

An important part of the magnetosphere is the magnetotail. The magnetotail is the part of the magnetosphere that extends away from the Sun, in the direction of the solar wind flow. Inside the magnetotail is the plasma sheet region, which is full of charged particles (plasma). The plasma sheet is important because it is the source region for the particles that get into the inner magnetosphere, creating the current that causes geomagnetic storms.

MIT researchers and colleagues have demonstrated a way to precisely control the size, composition, and other properties of nanoparticles key to the reactions involved in a variety of clean energy and environmental technologies. They did so by leveraging ion irradiation, a technique in which beams of charged particles bombard a material.

They went on to show that nanoparticles created this way have superior performance over their conventionally made counterparts.

“The materials we have worked on could advance several technologies, from fuel cells to generate CO₂-free electricity to the production of clean hydrogen feedstocks for the chemical industry [through electrolysis cells],” says Bilge Yildiz, leader of the work and a professor in MIT’s Department of Nuclear Science and Engineering and Department of Materials Science and Engineering.

Once inflation comes to an end, and all the energy that was inherent to space itself gets converted into particles, antiparticles, photons, etc., all the Universe can do is expand and cool. Everything smashes into one another, sometimes creating new particle/antiparticle pairs, sometimes annihilating pairs back into photons or other particles, but always dropping in energy as the Universe expands.

The Universe never reaches infinitely high temperatures or densities, but still attains energies that are perhaps a trillion times greater than anything the LHC can ever produce. The tiny seed overdensities and underdensities will eventually grow into the cosmic web of stars and galaxies that exist today. 13.8 billion years ago, the Universe as-we-know-it had its beginning. The rest is our cosmic history.

In 1973, physicist Phil Anderson hypothesized that the quantum spin liquid, or QSL, state existed on some triangular lattices, but he lacked the tools to delve deeper. Fifty years later, a team led by researchers associated with the Quantum Science Center headquartered at the Department of Energy’s Oak Ridge National Laboratory has confirmed the presence of QSL behavior in a new material with this structure, KYbSe₂.

QSLs—an unusual state of matter controlled by interactions among entangled, or intrinsically linked, magnetic atoms called spins—excel at stabilizing quantum mechanical activity in KYbSe₂ and other delafossites. These materials are prized for their layered triangular lattices and promising properties that could contribute to the construction of high-quality superconductors and quantum computing components.

The paper, published in Nature Physics, features researchers from ORNL; Lawrence Berkeley National Laboratory; Los Alamos National Laboratory; SLAC National Accelerator Laboratory; the University of Tennessee, Knoxville; the University of Missouri; the University of Minnesota; Stanford University; and the Rosario Physics Institute.